Three-phase Circuits
Article shared by: In this article we will discuss about: 1. Introduction to Three-Phase AC Circuits 2. Generation of 3-Phase EMF in AC Circuits 3. Phase Sequence 4. Conversion of Balanced Load System from Star to Delta and Vice-Versa 5. Balancing Parallel Loads.
Contents:. Introduction to Three-Phase AC Circuits. Generation of 3-Phase EMF in AC Circuits.
Phase Sequence in Three-Phase AC Circuits. Conversion of Balanced Load System from Star to Delta and Vice-Versa. Balancing Parallel Loads in 3 Phase AC Circuit 1. Introduction to Three-Phase AC Circuits: The type of alternating currents and voltages discussed so far in the book are termed as single phase currents and voltages as they consist of a single alternating current and voltage waves. Single phase systems involving single phase currents and voltages are quite satisfactory for domestic applications. Even the motors employed in domestic applications are mostly single phase, for example, motors for mixers, coolers, fans, air-conditioners, refrigerators.
However, the single phase system has its own limitations and, therefore, has been replaced by polyphase system. For supplying power to electric furnaces 2-phase system is generally employed. Six phase system is usually employed in connection with converting machinery and apparatus. For general supply three-phase system is universally used. For generation, transmission and distribution of electric power 3-phase system has been universally adopted.
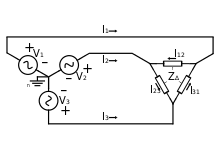
The two-phase supply and six-phase supply are obtained from 3-phase supply. Polyphase system means the system which consists of numerous (poly means numerous or multiple) windings or circuits (phase means winding or a circuit). A polyphase system is essentially a combination of several single phase voltages having same magnitude and frequency but displaced from one another by equal angle (electrical), which depends upon the number of phases and can be determined from the following relation: Electrical displacement = 360 electrical degrees/Number of phases (7.1) The above relation does not hold good for two phase windings, which are displaced by 90 electrical degrees apart. A supply system is said to be symmetrical when several voltages of the same frequency have equal magnitude and are displaced from one another by equal time angle. 3-phase, 3-wire or 4-wire supply system will be symmetrical when the line to line voltages are equal in magnitude and displaced in phase by 120 electrical degrees with respect to each other. Further in a four-wire system the voltage with respect to neutral of the three phase wires should be equal to one another in magnitude and displaced in phase by 120° with respect to each other.
Three Phase Circuits Theory
A 3-phase supply will be unbalanced when either of the three-phase voltages is unequal in magnitude or the phase angle between these phases is not equal to 120°. A load circuit is said to be balanced when the loads (impedances) connected in various phases are same in magnitude as well as in phase.
Any three phase load in which the impedances in one or more phases differ from the impedances of other phases is called an unbalanced three-phase load. In case one phase out of three phases of a 3-phase supply connected to the 3-phase load is not available, then such a condition is called the single phasing. Merits and Demerits of Polyphase System over Single Phase System: The advantages of a polyphase system over single phase system are enumerated below: (i) In a single phase circuit the power delivered is pulsating.
Even when current and voltage are in phase, the power is zero twice in each cycle, and when the current leads or lags behind the voltage, the power is negative twice and zero four times during each cycle. This is not objectionable for lighting and small motors but with large motors it causes excessive vibrations.
In polyphase system the total power delivered is constant if loads are balanced though the power of any one phase or circuit may be negative. So polyphase system is highly desirable particularly for power loads. (ii) The rating of a given machine increases with the increase in number of phases. For example output of a 3-phase motor is 1.5 times the output of a single phase motor of same size. (iii) Single phase induction motors have no starting torque and so it is necessary to provide these motors with an auxiliary means of starting, but in case of three-phase motors except synchronous motors, there is no need of providing an auxiliary means for starting. (iv) Power factor of a single-phase motor is lower than that of a polyphase motor of the same rating (output and speed).
The efficiency of a polyphase motor is also higher than that of a single-phase motor. (v) Three phase-system requires 3/4th weight of copper of that required by single-phase system to transmit the same amount of power at a given voltage and over a given distance. (vi) Rotating magnetic field can be set up by passing polyphase currents through stationary coils, (vii) Polyphase system is more capable and reliable than single-phase system, and (viii) Parallel operation of polyphase alternators is simple as compared to that of single-phase alternators because of pulsating reaction in single phase alternators. However, three-phase operation is not as practical for domestic applications where motors are usually smaller than 1 kW and where lighting circuits supply most of the load. Commonly Used Polyphase System: Although there are several polyphase systems such as two- phase, three-phase but 3-phase system is invariably adopted because of its inherent advantages over all other polyphase systems.
The demand for two-phase system has almost disappeared because that does not have any advantage that is not equaled or surpassed by three-phase system in generation, transmission or utilization. Three- phase system is universally employed for generation, transmission and distribution of electric power. Two- phase supply and six-phase supply, when required, is obtained from 3-phase supply. Systems with number of phases, more than three, increase complexity and cost of transmission and utilisation hardware and become uneconomical. Knowledge of 3-phase power systems is, therefore, essential for understanding of power technology. Fortunately, the basic circuit technique used in solving single phase circuits are directly applicable to 3-phase circuits because three phases are identical and one phase represents the behaviour of all the above.
In this article we will discuss only 3-phase systems. Generation of 3-Phase EMF in AC Circuits: When three coils fastened rigidly together and 120° (electrical) apart rotate about the same axis in a uniform magnetic field, the induced emf in each of them will have a phase difference of 120° or 2/3 π radians. Consider three identical coils a 1a 2, b 1b 2 and c 1c 2 mounted on the same axis but displaced from each other by 120° rotating in counter-clockwise direction in a bipolar magnetic field, as shown in Fig. Here a 1, b 1 and c 1 are the start terminals and a 2, b 2, and c 2, are the finish terminals of the three coils. When the coil a 1a 2 is in position AB the induced emf in this coil is zero and is increasing in positive direction, the coil b 1b 2 is 120° behind coil a 1a 2 so the emf induced in this coil is approaching its maximum negative value and the coil c 1c 2, is 240° behind coil a 1a 2 so as the emf induced in the coil has passed its positive maximum value and is decreasing. Since each coil being identical has an equal number of turns and is wound with the wire of the same type and same x-section, the induced emfs in each of the coils are of same magnitude.

The induced emf in each coil is also of same frequency and same waveform (sinusoidal in this case) but displaced from each other by 2π/3 radians or 120°, as illustrated in Fig. 7.1 (b) by waveforms.
Accordingly, the instantaneous values of the emfs induced in coils a 1a 2, b 1b 2 and c 1c 2 may be given as: if t = 0 corresponds to the instant when the voltage or emf of coil a 1a 2 passes through zero and increases in positive direction. Double Subscript Notation: The solution of problems involving circuits and systems containing a number of voltages and currents is simplified and less susceptible to error if the voltage and current phasors are designated by some systematic notation.
The double subscript notation is a very useful concept from this point of view. In this notation two letters are placed at the foot of the symbol for voltage or current, the order in which the subscripts are written, indicates the direction in which the voltage acts or current flows. For example, if a voltage in a circuit acts in such a direction as to cause a current to flow from A to B, the positive direction of voltage is from A to B, and the voltage may be represented by V AB or E AB, the order of the subscripts denoting that the voltage or emf is acting from A to B. If the voltage is indicated by V BA or E BA it means that point B is positive w.r.t.
Point A (during its positive half-cycle) and the voltage causes the current to flow from B to A i.e., V BA or E BA indicates that voltage or emf acts in a direction opposite to that in which V AB or E AB acts. So V BA = – V AB (7.3) Similarly I AB indicates that current is flowing from A to B but I BA indicates that current is flowing in direction from B to A – i.e., I BA = – I AB. Phase Sequence in Three-Phase AC Circuits: Phase sequence is the order or sequence in which the currents or voltages in different phases attain their maximum values one after the other. 7.1 (a) three coils a 1a 2, b 1b 2, and c 1c 2 rotating in anti-clockwise direction are shown. Since the coil b 1b 2, is 120° behind coil a 1a 2 and coil c 1c 2 is 240° behind coil a 1a 2, therefore, first coil a 1a 2, attains maximum or peak value of induced emf, the coil b 1b 1 attains maximum or peak value of induced emf, when the coils rotate further 120° (electrical) and coil c 1c 2 attains peak value of induced emf when the coils rotate through 240° (electrical).
Since the induced emfs in three coils a 1a 2, b 1b 2 and c 1c 2, attain maximum values in order a, b, c, phase sequence is, a b c. If the direction of rotation of the coils is reversed i.e., clockwise, the three-phase emfs would attain their maximum values in order a, c, b and, therefore, phase sequence would be acb. Since the coils can be rotated in clockwise or in counter-clockwise direction, there can be only two possible phase sequences. The knowledge of phase sequence of a 3-ɸ supply is essential for making connections for alternators and transformers to operate them in parallel. Reversal of phase sequence of a 3-phase alternator which is to be paralleled with another similar alternator may cause extensive damage to both the machines.
3 Phase Delta Vs Wye
The direction of rotation of an induction motor depends on the phase sequence. If the phase sequence is reversed by interchanging any two terminals of the 3-ɸ supply, the motor would rotate in the opposite direction. The phase sequence of the voltage applied to the load, in general, is determined by the order in which three phase lines are connected. In the case of 3-phase unbalanced loads, the effect of reversal of phase sequence, in general causes a completely different set of values of the currents. So while working on such systems it is essential to specify the phase sequence clearly to avoid unnecessary confusion. Conversion of Balanced Load System from Star to Delta and Vice-Versa: Any balanced star-connected system can be completely replaced by an equivalent delta-connected system or vice-versa because of their relationships between phase and line voltages and currents.
For example, a balanced star-connected load having an impedance of magnitude Z with a power factor cos ɸ (or Z ∠ɸ) in each phase can be replaced by an equivalent delta-connected load having an impedance of magnitude 3Z and power factor cos ɸ (i.e. 3Z ∠ɸ) in each phase. This may be established as follows: Now in equivalent delta-connected system for the same line values of voltage and current as in case of star-connected system: 5. Balancing Parallel Loads in 3 Phase AC Circuit: A combination of balanced 3- ɸ loads connected in parallel may be solved by any one of the following methods: 1. All the given loads may be converted into either equivalent Y or Δ-connected loads and then combined together according to the law governing parallel circuits. Alternative method is of working out volt-amperes. The real power and reactive power of various loads may be added arithmetically and algebraically respectively in order to give total volt-amperes according to expression: Where P is the power is watts (or kW), Q is the reactive power in reactive volt-amperes (or kVAR) and S is the volt-amperes (or kVA) Example: A 3-phase star-connected 1,000 V alternator supplies power to 500 kW delta-connected induction motor.
If the motor power factor is 0.8 lagging and its efficiency 0.9, find the current in each alternator and motor phase. Solution: Motor input, P = Motor output/Motor efficiency = 500/0.9 = 555.55 kW Motor power factor, cos ɸ = 0.8 (lagging).
Cos φ = power factor φ = Power factor angle Also, learn about:. Example 1: A 240 volts, three phase circuits draws a phase current of 3.5 A at a power factor of 0.9. Find the power in watts.
Solution: P = 1.73. V p.I p cos φ = 1.73. 240. 3.5 A.
0.9 = 1307 watts Example 2: A 200-volt three phase circuit draws a phase current of 15 amps. The power factor of the circuit is 0.9.
Find the power. Solution: P = 1.73. V p.I p cos φ = 1.73. 200.
15A. 0.9 = 4671 watts Other useful formulae:.